Crystal Structures
- Engineering

- Nov 27, 2020
- 3 min read
Updated: Apr 23, 2021
In this notes sheet:
Understanding the molecular structure of a material is crucial in understanding how that material behaves. Many of its physical properties are directly caused and explained by its crystalline structure (known as a lattice). We define this as:
A crystalline lattice is a regular repeating pattern of atoms or molecules.
An amorphous material is one that does not display a crystalline structure. Instead, the structure is random – examples include some polymers and ceramics.
Some other polymers are known as semi-crystalline
The Hard Sphere Model
The Hard Sphere Model allows us to model atoms or molecules as, you guessed it, hard spheres. There are a number of conditions for this to apply:
The outer electron shell must be full
The remaining electrons must be distributed rotationally symmetrically
Electron clouds of neighbouring atoms do not overlap much or at all
For the outer shell of electrons to be full, metals must lose electrons, while non-metals must gain electrons. This means they are actually ions.
Packing in Two Dimensions
It is impossible to arrange circles in such a way that there are no unfilled spaces between them:
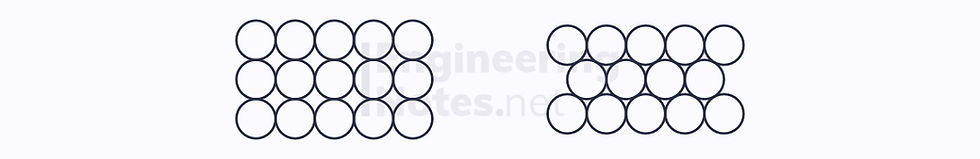
Squares, rectangles, triangles, and hexagons, however, can be arranged into a complete lattice:

The primitive unit cell is the smallest cell that can be regularly repeated throughout the structure – the hexagon can be split into six equilateral triangles.
The unit cell is the easiest cell to repeat: the hexagonal is more practical, as it does not require rotating, whereas the triangle does.
The two arrangements of circles above can, however, be modelled as square and rectangular patterns respectively, where the centre of an atom is at each vertex:
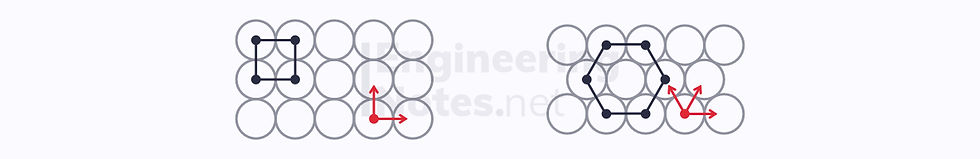
Shown in red on the diagrams are the close-packed directions of each structure. These are the lines along which ions continuously touch (the line never passes through empty space). As you can see:
The square lattice has two close-packed dimensions
The hexagonal lattice has three close-packed directions
Since the hexagonal lattice is more densely packed, it is known as the close-packed arrangement.
Packing in Three Dimensions
If we look at the same arrangements in 3D, is clear that, as well as close-packed directions, there are close-packed planes. These are planes in which all the ions are arranged in a hexagonal lattice.
There are four typical unit cells in three dimensions:
Simple Cubic
This is simply a 2D square lattice in 3D:

1 atom per unit cell (⅛ on each vertex)
6 nearest neighbours (each ion touches six others - also known as the coordination number)
Lattice parameter: a = 2r
No close-packed planes
No slip systems
Atomic packing factor: 52.4%
Body-Centred Cubic (BCC)
In the simple cubic cell above, there is a gap in the centre. When the outer atom eights are moved apart enough to fit another atom in the middle, you have the BCC arrangement:
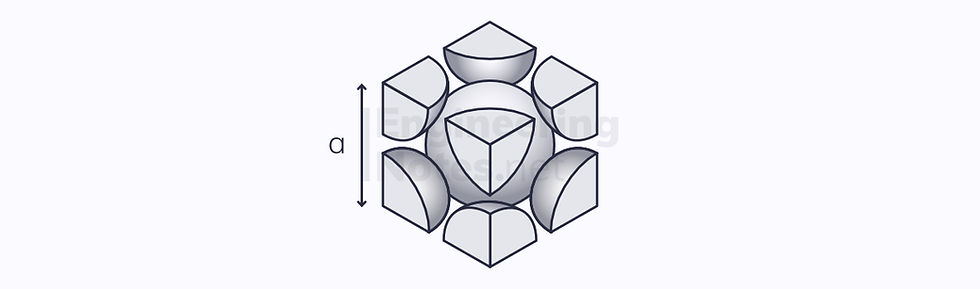
2 atoms per unit cell (⅛ at each vertex, one in the centre)
8 nearest neighbours
Lattice parameter a = ⁴/₃ √3 r
No close-packed planes
2 close-packed directions
No slip systems
Atomic packing factor: 68.2%
Face-Centred Cubic (FCC)
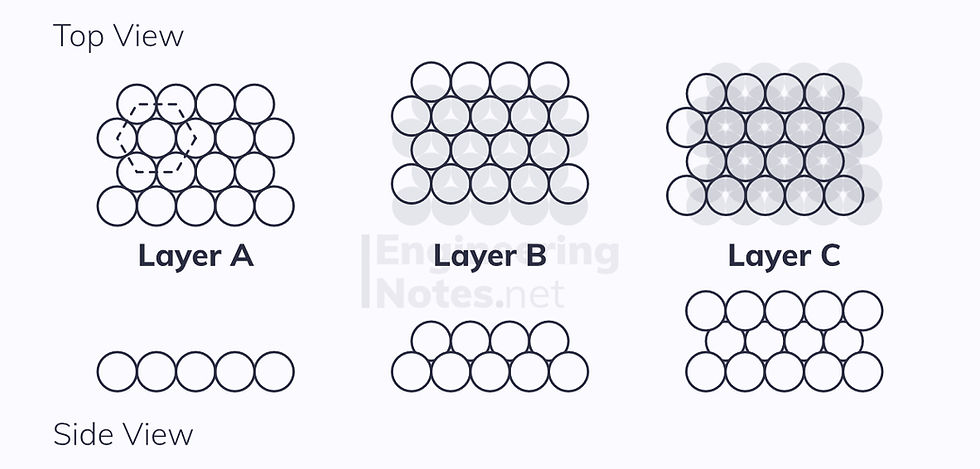
This is the most densely packed cubic arrangement. In this instance, there are three close-packed planes stacked on top of one another, in an ABC arrangement:
Again, the unit cell is a cube, but the atoms on the vertices are further apart. This allows 4 half atoms to fit in the centre:
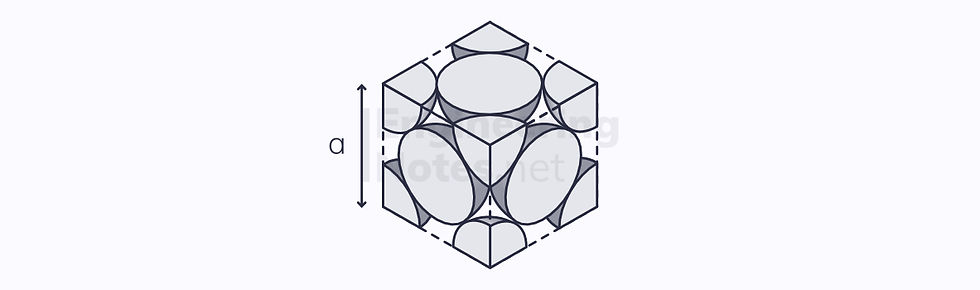
4 atoms per unit cell (⅛ on each vertex, ½ on each side)
12 nearest neighbours
Lattice parameter a = √2 r
4 close-packed planes
3 close-packed directions
12 slip systems
Atomic packing factor: 74%
Hexagonal Close-Packed (HCP)
This is where there are two close-packed planes stacked one on top of the other, this time in an ABAB arrangement:

This leads to a hexagonal unit cell:
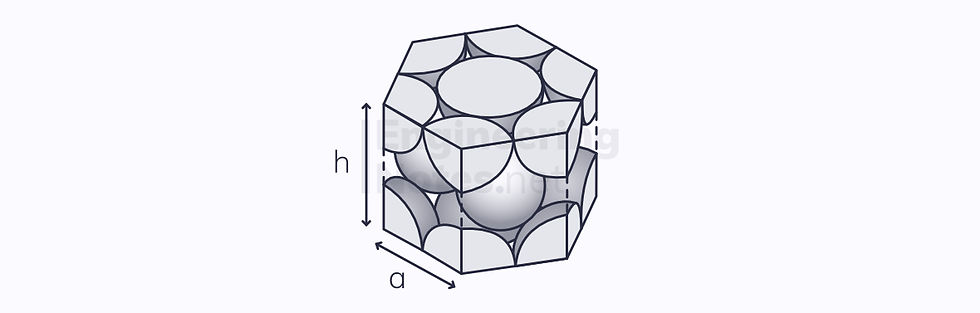
6 atoms per unit cell (⅙ on each vertex, ½ on top and bottom, 3 in centre)
12 nearest neighbours
Lattice parameter: a = 2r
1 non-parallel close-packed plane
3 close-packed directions
3 slip systems
Atomic packing factor: 74%
The height of the hexagonal close-packed unit cell is given as:

Note that HCP and FCC both have the same atomic packing factor, 74%
Atomic Density
If we know the atomic radius, mass and the type of unit cell, we can estimate the density of a material:
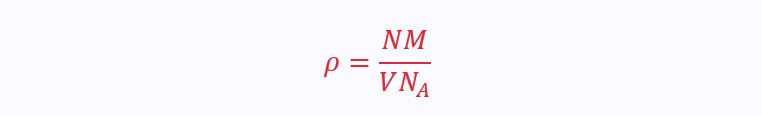
N is the number of atoms per unit cell
M is the atomic mass
V is the volume of the unit cell
N(A) is Avogadro’s Number, 9.02x10²³

Comments