Thermal Physics

The temperature of a substance is defined by the amount of internal energy it has. Generally, warmer substances will transfer thermal energy to cooler substances until they are the same temperature - this is known as thermal equilibrium.

In every day life, we use either °C or °F to measure temperature. The degree, °, however, shows that the temperature is relative to a substance-specific standard. Degrees centigrade are related to water, with 0 °C being its freezing point and 100 °C its boiling point. Degrees Fahrenheit is jut a mess with water being liquid between 32 °F and 212 °F.

In physics, using a relative temperature scale is not practical. Instead, absolute temperature is used, the units of which are Kelvin, K. Zero Kelvin is not relative to any particular substance - rather it is the absolute minimum possible internal energy (where particles have no kinetic energy at all, so do no vibrate)

T = θ + 273 Absolute temperature (in K) = Relative temperature (in °C) + 273

• A change of 1 K is the same as a change of 1 °C

• T is used for absolute temperature, θ for relative

• Absolute zero is -273 °C

Solids, Liquids & Gasses

The kinetic model explains the three phases of matter:

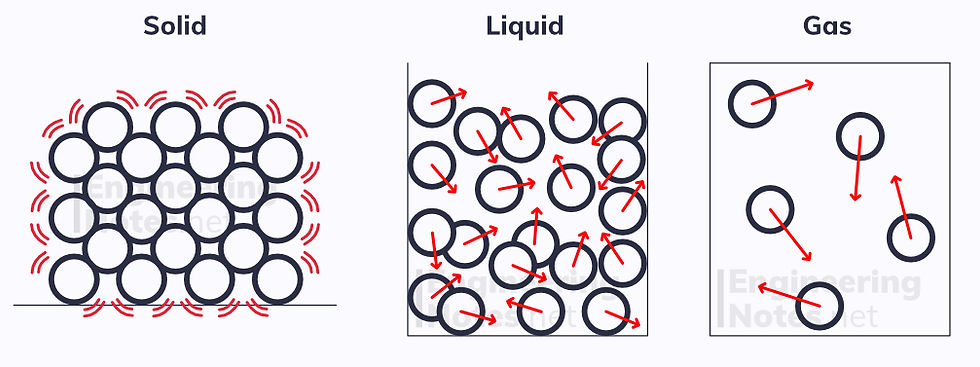
Solids

Solids have their particles arranged in a regular pattern, all touching one another. They vibrate around fixed positions, and are held together by strong electrostatic attractive forces. The greater the thermal energy, the greater kinetic energy the particles have, so they vibrate faster.

Liquids

The particles in a liquid are further spaced apart, but generally still touching. They are not fixed into a regular arrangement, but are free to move around - this allows liquids to flow. Due to this, liquids adapt to the shape of a container. The greater the thermal energy, the faster the particles move (as they have more KE).

Gasses

In a gas, the particles are completely free to move, and do so at a high speed. They fill the space they are confined to, and collide with the walls of the container. This is why gasses exert pressure on a container. Again, the more thermal energy, the more KE the particles have.

Brownian Motion

Liquids and gasses are grouped together as fluids, because their particles are free to move. Their movement is random and in a zigzag (caused by collisions with other particles and boundaries). This is known as Brownian motion, and supports the kinetic model.

This can be demonstrated with pollen particles suspended in water, or smoke particles in air, viewed through a microscope.

Internal Energy

All matter has energy contained within it, known as internal energy.

Internal energy is the sum of the randomly distributed kinetic and potential energies of the particles in a system

The kinetic energy of a particle is dependent on its mass and speed. As seen above, kinetic energy is proportional to temperature. At 0 K, the particles have 0 kinetic energy.

The potential energy is down to the electrostatic interactions between different particles. This is never 0, even at absolute zero.

Therefore at absolute zero, internal energy is at its minimum but not zero. As temperature increases, so does internal energy.

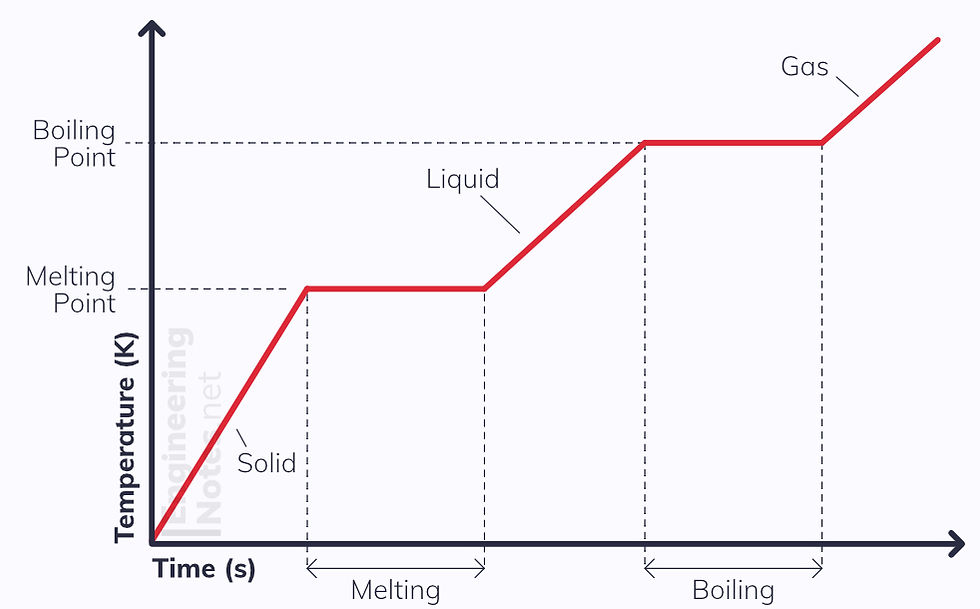
Changes of Phase

During a change of phase, the internal energy of the substance changes. This is due to a change in potential energy not a change in kinetic energy.

When the substance changes phase, its temperature stays the same but its molecular structure changes.

For example, ice melts at 273 K, or 0 °C. In the process, the potential energy of the substance increases, but both the ice pre-melt and water post-melt are at 273 K, 0 °C.

Similarly when water boils, both the water immediately before evaporating and the gas immediately after are at 100 °C because the kinetic energy is constant throughout changes of phase.

This often causes confusion because changes of state are seen to be brought on by a change in temperature, but this is not strictly the case.

Thermal Properties

All materials have certain thermal properties that are fixed to that material.

Specific Heat Capacity

Specific heat capacity, c, is defined as the amount of energy required to raise the temperature of 1 kg of a substance by 1 K (or 1 °C). It is a fixed property of a substance/material.

E = mcΔθ Energy change = mass x specific heat capacity x change in temperature (in K)

Specific Latent Heat

The specific latent heat of a substance, L, is defined as the energy required to change the phase of the substance per unit mass while at a constant temperature.

L = E/m Specific latent heat = energy supplied / mass

There are two forms of specific latent heat:

• Specific Latent Heat of Fusion is the energy required to change a substance from a solid to a liquid

• Specific Latent Heat of Vaporisation is the energy required to change a substance from a liquid to a gas

The specific latent heat is the change of potential energies that occurs in a substance when it changes phase, increasing its internal energy. Remember that the kinetic energies remain constant.

Ideal Gasses

When working with gasses, using mass as a measurement is not practical (a kg of air is over 150,000 teaspoons!). Instead, we use the number of particles, often measured in moles.

One mole contains 6.03 E23 particles

This is known as Avogadro's constant.

• To find the number of moles, n, multiply the number of particles by Avogadro's constant.

• To find the number of particles, N, divide the number of moles by Avogadro's constant.

Small n for the smaller number (number of moles), big N for the bigger number (number of particles)

Kinetic Theory for Ideal Gasses

We use the kinetic theory (see above) for gasses. This means we make certain assumptions, which is why we call them ideal gasses:

• The gas contains a large number of molecules

• The gasses follow Brownian Motion - move at random directions with random speeds

• The molecules occupy a negligible volume compared to the volume of the gas

• All collisions with other molecules or the container walls are perfectly elastic, meaning that both momentum and kinetic energy is conserved

• The collisions are of negligible duration compared to the time between collisions

• Only forces during collisions are worth noting (electrostatic interactions are negligible)

When the gas molecules collide with the walls of the container, they change direction. This means there has been a change in momentum of -2mu, where m is the mass of the molecule and u its speed. Before the collision, its velocity is +u, after the collision it is -u (-u - +u = -2u). The change in momentum is the same as the impulse, Ft exerted on the wall. This impulse (due to Newton's third law) exerts a very small force on the wall, but many many collisions are taking place. The force of all of these collisions combined is what exerts a pressure on the walls, pressurising the container.

Gas Laws

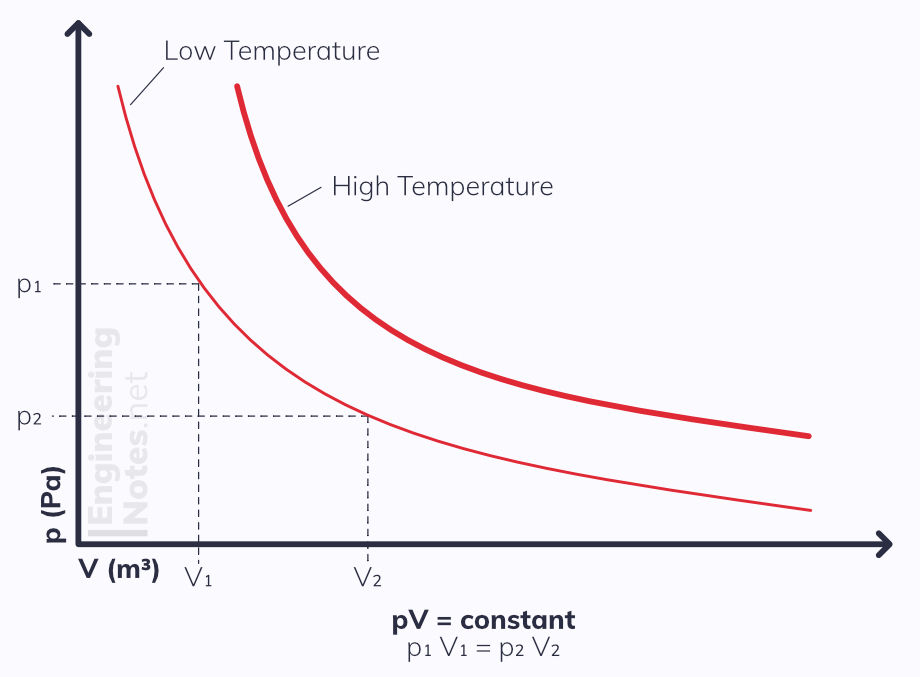
Boyle's Law

Boyle's law states that when temperature and mass of a gas remain constant, the pressure of the gas, p, is inversely proportional to its volume, V, and so pV is constant.

p ∝ 1/V pV = constant

The Pressure Law

The Pressure law states that when the volume and mass of an ideal gas remain constant, the pressure, p, is directly proportional to its absolute temperature in Kelvin, K.

p ∝ T p/T = constant

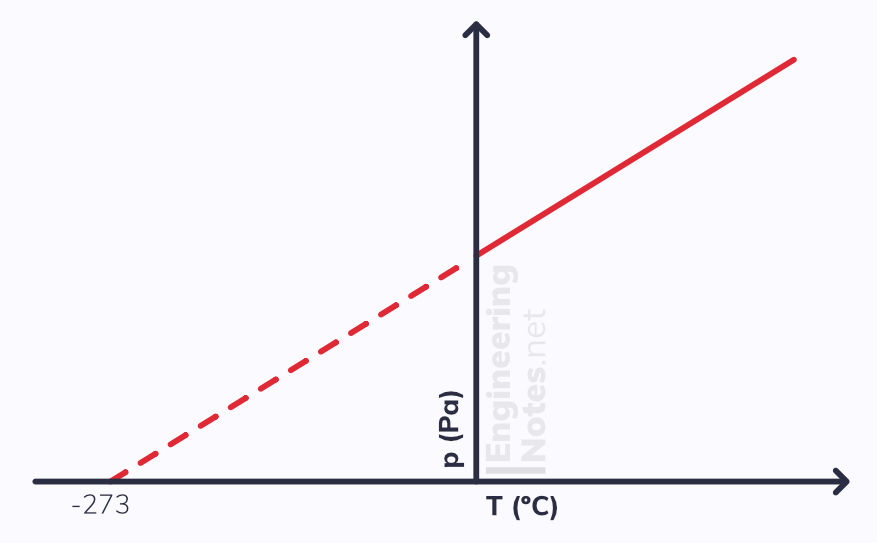
This can be used to workout an estimate for absolute zero by submerging a flask of air in a water bath, and connecting it with an air tight tube to a pressure gauge. As the temperature of the water increases, so does the temperature of the air. This, in turn, increases the pressure inside it. Plotting a graph of pressure against temperature shows direct proportion as it will have a straight line of best fit. The line does not go through the origin, but extrapolating it backwards to meet the x-axis will give an estimate for absolute zero.

The two equations above can be combined:

pV/T = constant

For one mole of an ideal gas, the constant in this equation is the molar gas constant, R, 8.31 J/K/mol

Adding this to the equation gives pV/T = R. For when you have a different number of moles, n:

pV/T = nR

This is known as the ideal gas equation.

Charles's Law

Charles's Law states that when the pressure of an ideal gas is fixed, the volume, V, of the gas is directly proportional to its absolute temperature, T.

Root Mean Square (r.m.s.) Speed

When looking at the energy of a gas, we need to know the average velocity of all the particles in the gas. However, we cannot calculate this, because velocity is a vector quantity, and so has direction: all the particles are travelling in different directions so cancel out. This means the actual average velocity is 0 m/s.

Instead, we use root mean square speed, the square root of the mean of the square of the velocities of the gas particles.

√mean of square of speed √c̅²

The units of c.m.s are m²s-²

The mean square speed (not rooted) is used in the pressure & volume equation:

pV = 1/3 Nm c̅ ²

Where p is the pressure, V the volume, N the number of particles in the gas, m the mass, and c̅ ² the mean square speed.

• If you have the number of moles, multiply by Avogadro's constant to find the number of particles.

The Boltzmann Constant

The Boltzmann constant, k, is equal to the molar gas constant divided by Avogadro's constant:

k = 1.38 E-23

This can be substituted into the ideal gas equation:

pV = NkT

Kinetic Energy of Gasses

Combing the above equations relates average kinetic energy to absolute temperature:

1/3 Nm c̅ ² = pV = NkT

1/3 Nm c̅ ² = NkT Equating the equations

1/3 m c̅ ² = kT Cancelling the number of particles, N

2/3 x 1/2 m c̅ ² = kT Rewriting 1/3 as 3/2 x 1/2

1/2 m c̅ ² = 3/2 kT Dividing by 2/3

This is now in the form of the kinetic energy equation, KE = 1/2 mv². Therefore this is the equation for mean kinetic energy of a gas:

KE = 1/2 m c̅ ² = 3/2 kT

Internal Energy of Ideal Gasses

Internal energy is the sum of potential and kinetic energies in a system. One of the ideal gas assumptions, however, is that the electrostatic potential energy is negligible - this means that the internal energy of an ideal gas is the sum of kinetic energies in the particles.