Circles

Often when trying to find the equation of a circle, you will be given a line that intersects with the circle twice (it may be the diameter). To work out the circle from this you need to know the midpoint:

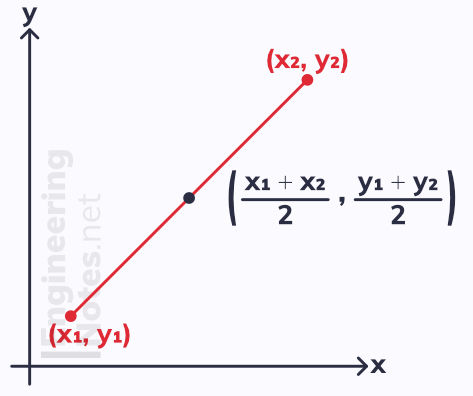
The perpendicular bisector of a cord will always pass through the centre of a circle. To find this, find the midpoint of the cord and find the equation of the line perpendicular to it (the negative reciprocal of the gradient)

Equation of a Circle

The points on a circle are all related through the equation of a circle.

For a circle with centre (0,0) and radius r, the equation is:

x² + y² = r²

For a circle with centre (a, b) and radius r, the equation is:

(x-a)² + (y-b)² = r²

Expanded Form

Sometimes, the equation is given or needed in expanded form:

x² + y² + 2fx + 2gy + c = 0

• (-f, -g) is the centre of the circle

• √(f² + g² -c) is the radius

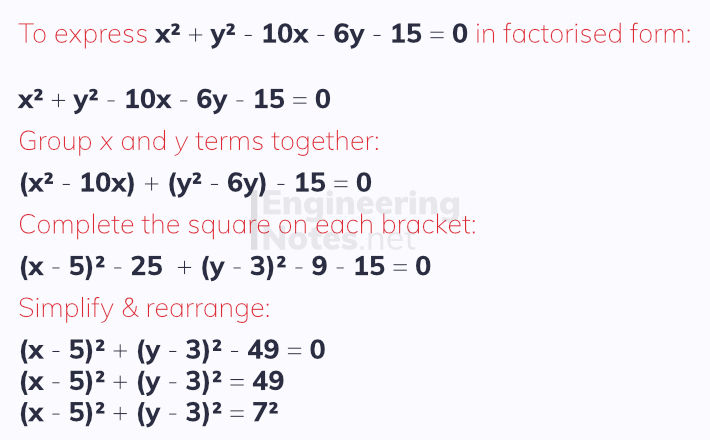
To go back from expanded form to the standard factorised form for an equation of a circle, it is quickest to complete the square:

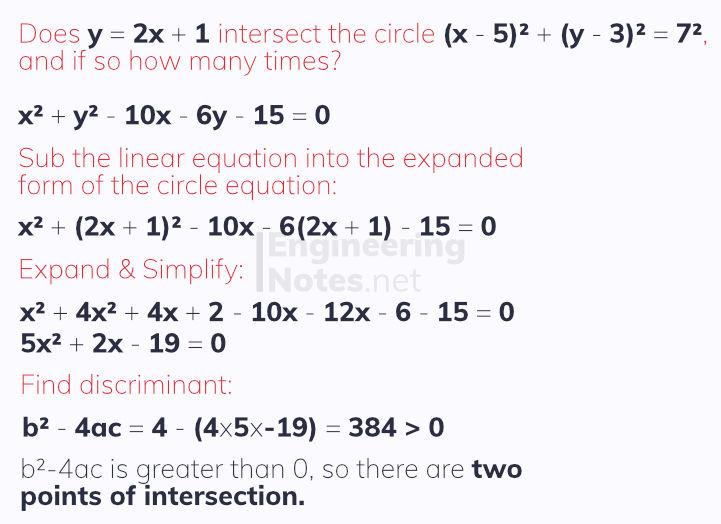
Intersecting Lines & Circles

A straight line can intersect a circle once, twice, or not at all:

To find out how many intersections there are between a circle and a straight line without sketching it, you can solve simultaneously:

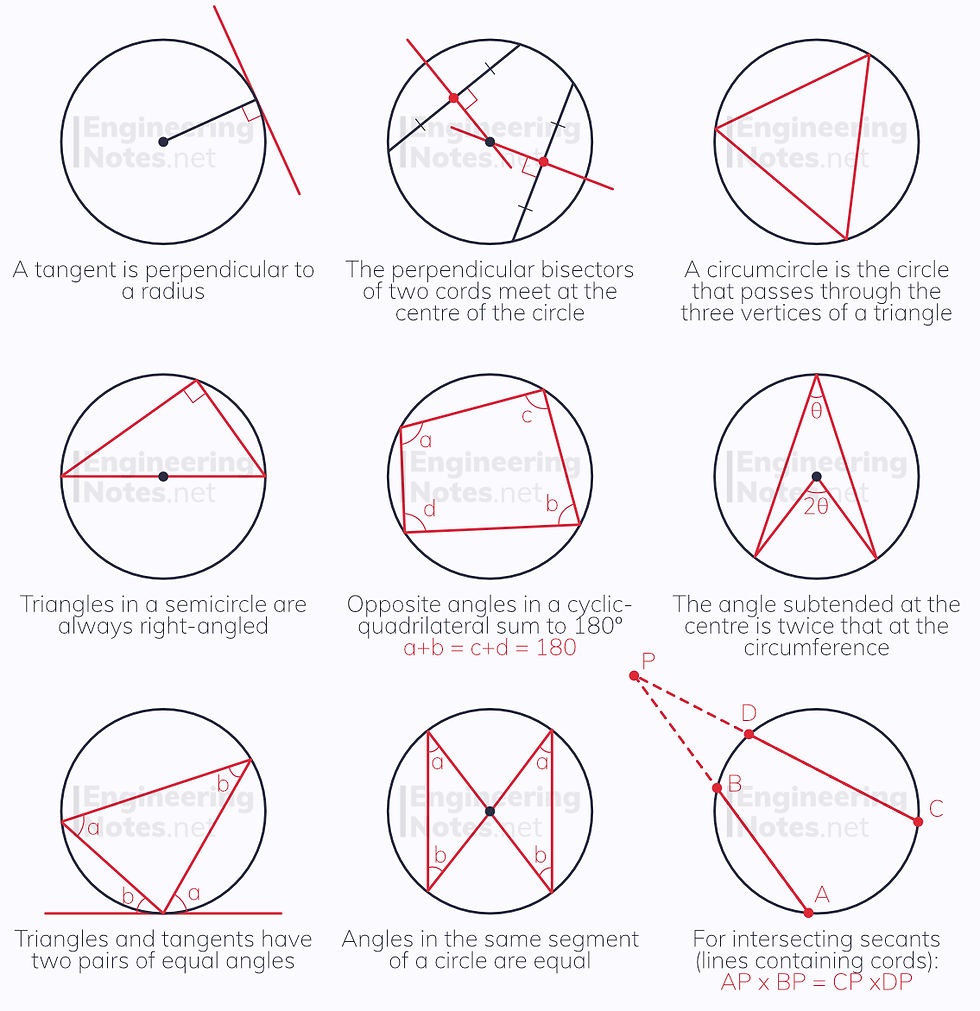
Circle Theorems