Integration

Before going too far down this notes sheet, ensure you're on top form for everything differentiation... else this will be harder than it needs to be!

Integration is the opposite of differentiation, and can be used to find the initial function form its derivative. For example, to find f(x) from f'(x), you integrate. It is also commonly used to find the area between a curve and the x-axis.

Integrating xⁿ

When differentiating xⁿ, you multiply by the power and then reduce the power by one. The reverse of this is to add one to the power and divide by this:

If f'(x) = xⁿ, then f(x) = (xⁿ⁺¹) / (n+1)

If there is a coefficient of x, you do the same but:

If f'(x) = kxⁿ, then f(x) = k(xⁿ⁺¹) / (n+1)

Note the difference between differentiating and integrating xⁿ:

• Just like when differentiating polynomials (functions with multiple terms), integrate one term at a time.

Definite & Indefinite Integrals

Integration is often noted using ∫ () dx, where the elongated S tells you to integrate the function in the brackets while the dx tells you to do so with respect to x. It is important to get this notation right.

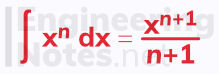
• When there are no limits with the ∫, the integral is called indefinite, because it can only give a function with an unknown y-intercept, c.

• If there are limits attached to the ∫, a and b, the integral is definite, as it produces a value for a defined interval [a, b].

Indefinite Integral Functions

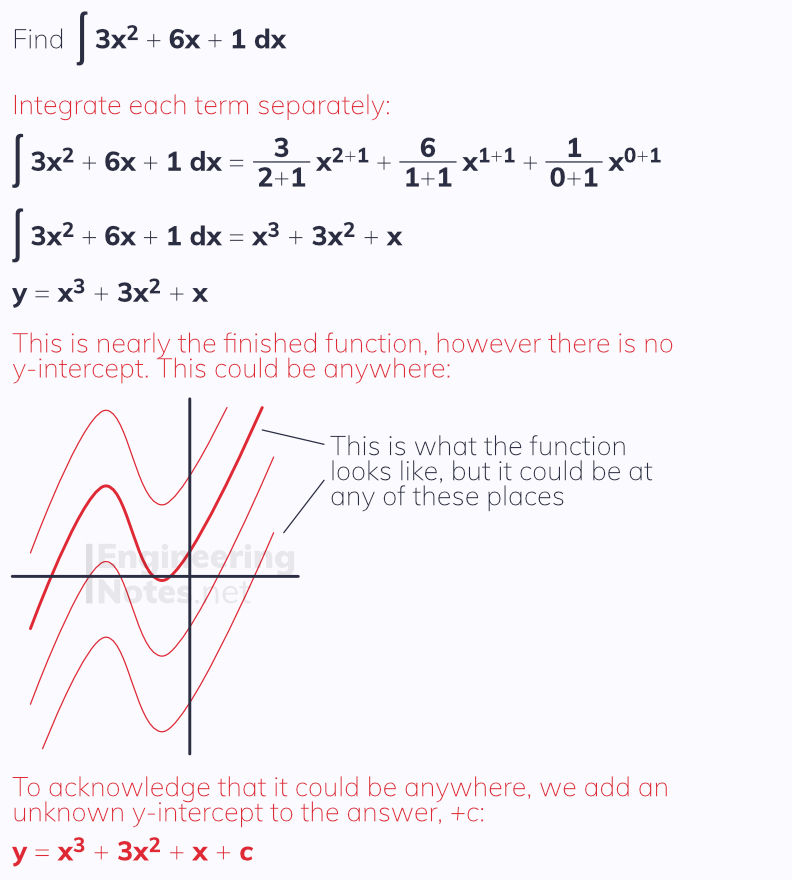
Unless you are given more information, indefinite integrals give an unspecific function. This means they produce a function but do not locate it - as in it could intersect anywhere on the y-axis. This is because when you differentiate a function, the y-intercept is not an x-term, so disappears. Therefore, when integrating the derivative, you have no idea where the y-intercept is, so we note it as an unknown, c.

You always need to add ' +c ' to unspecific integral functions, else it is not complete.

Finding the y-intercept, c

However, when you are given more information than just what to integrate, for example a coordinate that the function passes through, you can find the value of c, the y-intercept to find the particular integral:

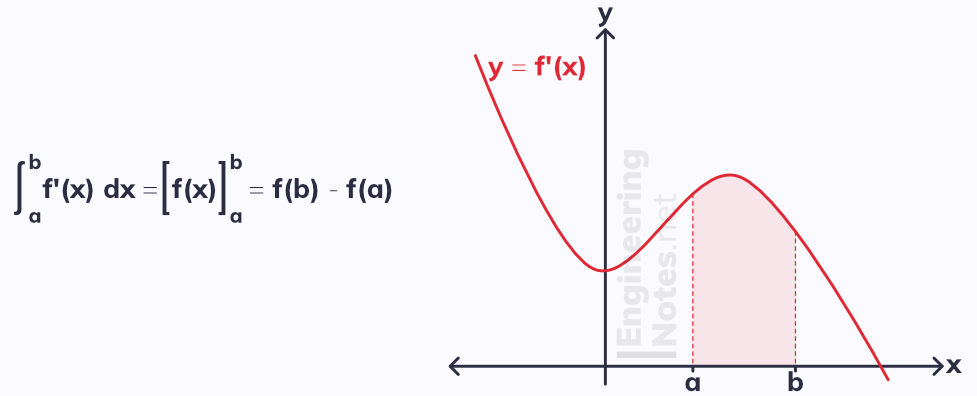
Areas under Graphs

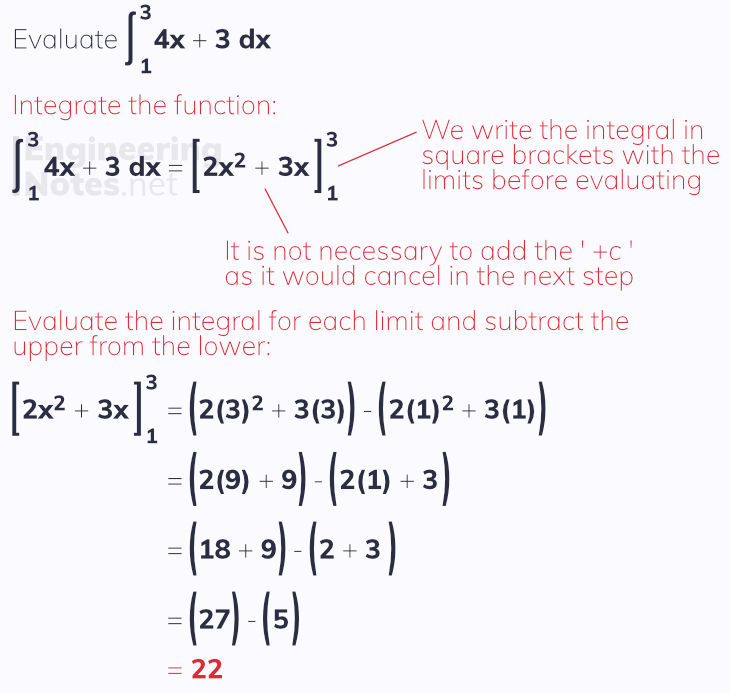
When given a definite integral, you integrate the function, and then evaluate it by substituting in the limits and subtracting the lower from the upper:

∫[a, b] f'(x) dx = f(b) - f(a)

For example, to evaluate 4x+3:

While differentiation is used to find the gradient of a function, integration is used to give the area enclosed by the graph and the x-axis and the limits.

Therefore, the example above is actually how to calculate the area under the line y = 4x + 3 between x=1 and x-3:

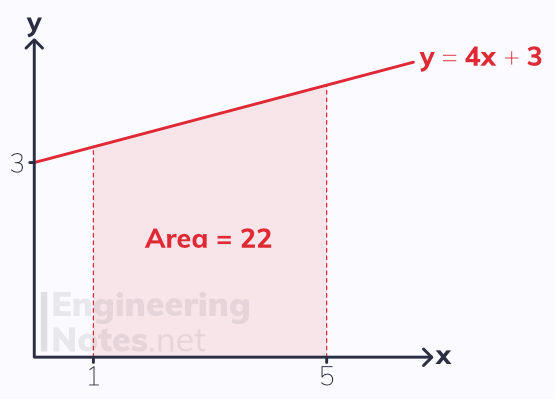
Evaluating definite integrals gives the area enclosed by the function, the limits and the x-axis.

Areas under the x-axis

If the graph is below the x-axis, the value will be negative.

Therefore, when there is a root in an interval, you need to find the root and split it up into two separate definite integrals and evaluate them separately.

• Find the x-coordinate of the root, r

• Evaluate two integrals, one on the interval [r, b] and one over [a, r]

• Add the positive integral to the modulus of the negative integral

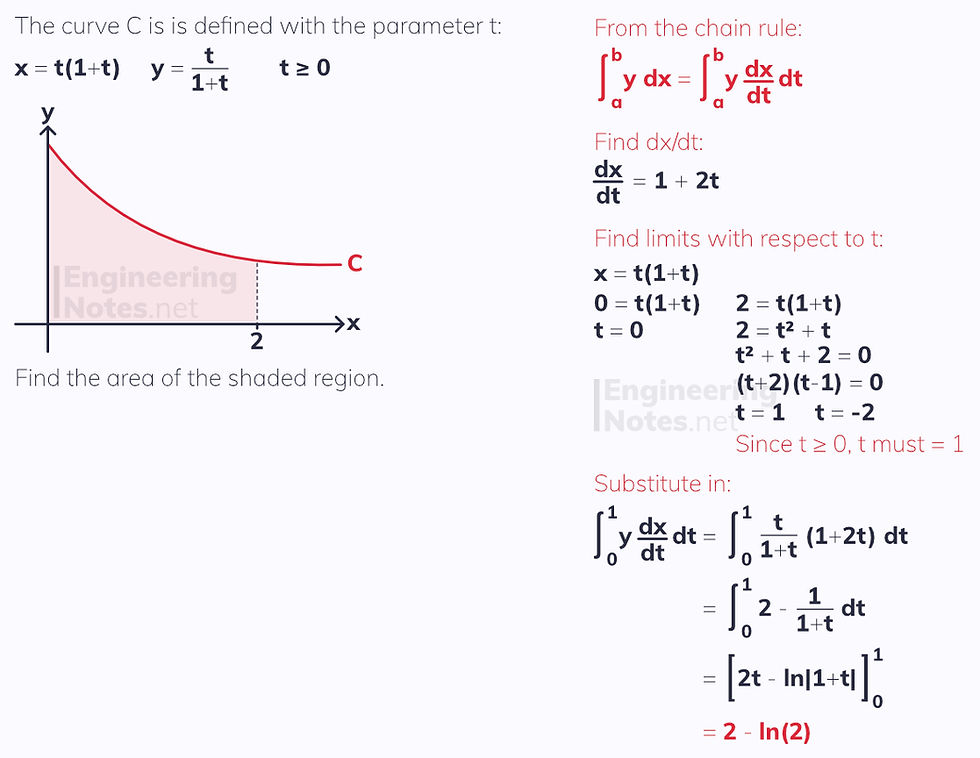
Area under Parametric Curves

• This requires the standard function for integrating 1/x - see below

It is not necessary to find the Cartesian form of a curve given as parametric to integrate it. Instead, we can use the chain rule to write dx as dx/dt, and integrate with respect to the parameter, t:

If x and y are given as functions of t, ∫y dx = ∫y dx/dt dt

• It is important to remember to change the limits into t, as you are integrating with respect to t.

Area Between Graphs

When trying to find the area enclosed by two or more graphs, it is easiest to split it up into the separate parts from each graph and the add or subtract accordingly.

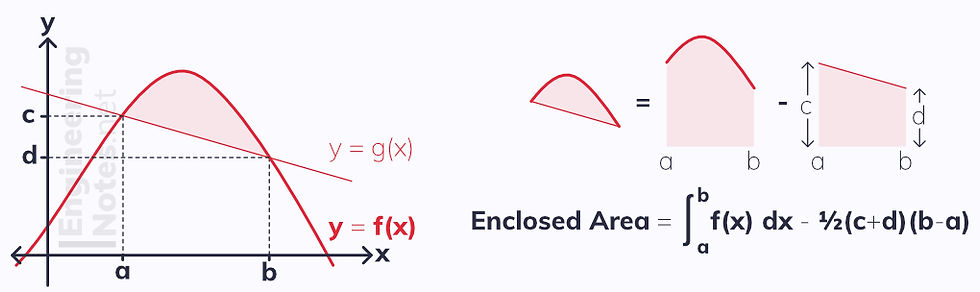
Areas between curves & lines

To find the area enclosed between a curve and a straight line, you need to find the area between each and the x-axis and then subtract one from the other. Often, you will have to use equations of triangle and trapezium area:

• Area of a triangle = ½ ab sinC

• Area of a trapezium = ½ (a+b)h

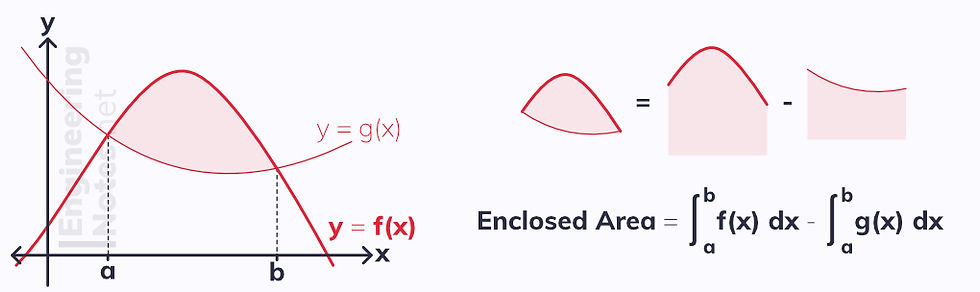
Areas between curves

To find the area between two curves, you use integration to calculate the area between each curve and the x-axis, and then subtract one from another.

Integrating Standard Functions

We have covered how to integrate xⁿ, but there are nine more standard functions you need to know:

∫ xⁿ dx = (xⁿ⁺¹) / (n+1) + c

∫ e ˣ dx = e ˣ + c

∫ 1/x dx = ln|x| + c

∫ cos(x) dx = sin(x) + c

∫ sin(x) dx = -cos(x) + c

∫ tan(x) dx = ln|sec(x)| + c

∫ sec²(x) dx = tan(x) + c

∫ cosec(x) cot(x) dx = -cosec(x) + c

∫ cosec²(x) dx = -cot(x) + c

∫ sec(x) tan(x) dx = sec(x) + c

∫ f'(ax+b) dx = f(ax+b) / a + c

Vital things to know

• The last standard function only works for functions in the form f(ax+b) and not for trigonometric functions like cos(ax+b).

• Only the trigonometric functions listed here can be integrated. For other functions, such as to integrate tan(x), you need to use trigonometric identities (see notes sheet on trigonometry) to get them into a form that can be integrated.

• Often, to get a function into a format that can be integrated you need to split it into partial fractions. Then, you can use the standard result for 1/x, or the reverse chain rule (see below)

Reverse Chain Rule

The reverse chain rule can be used when functions can be written in the form k f'(x) / f(x). The numerator must be an exact multiple of the derivative of the denominator for this to apply.

For example:

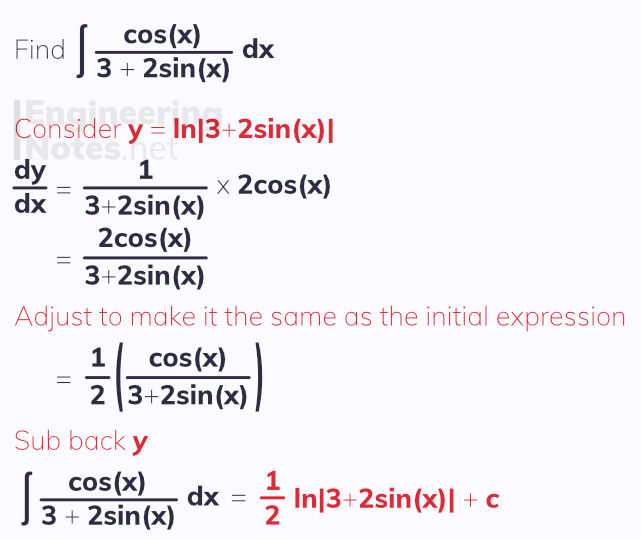
The reverse chain rule can also be used when functions can be written in the form k f'(x) ( f(x) )ⁿ.

For example:

• In both instances, adjustment may be required after trying the respective solutions.

Integration by Substitution

Often, you can make a complicated integral simpler by changing the variable. The difficult bit can be knowing what to use as your substitution, but in general:

• If there is a bracket to a high power, make this bracket the substitution

• In fractions, make the denominator your substitution

• If there is a root, make this the substitution

To integrate by substitution:

1. Write out your substitution as u

2. Rearrange this to make x the subject

3. Find du/dx

4. Rearrange this to make dx the subject

5. Manipulate the initial expression to replace dx with du and get it in terms of u only

6. Integrate in terms of u

7. Re-substitute u back to the initial form, and evaluate with limits

Do not evaluate limits while using the substitution, as the limits will be invalid and give the incorrect answers. Only evaluate once you have substituted back to x

Integration by Parts

This is the integration equivalent of the product rule used in differentiation, and is used when you need to integrate two functions that are multiplied together:

• where u and v are functions of x

This only works when the expression you are trying to integrate is in the form u dv/dx, so you need to choose which part to set as u and which as v.

• u will need to be differentiated

• v will need to be integrated

It is a good idea to set dv/dx as the part of the expression that is easiest to integrate

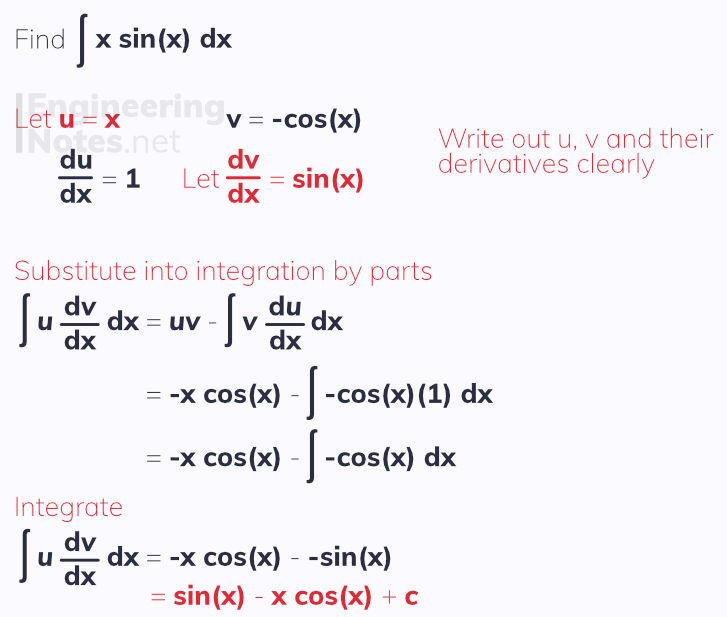
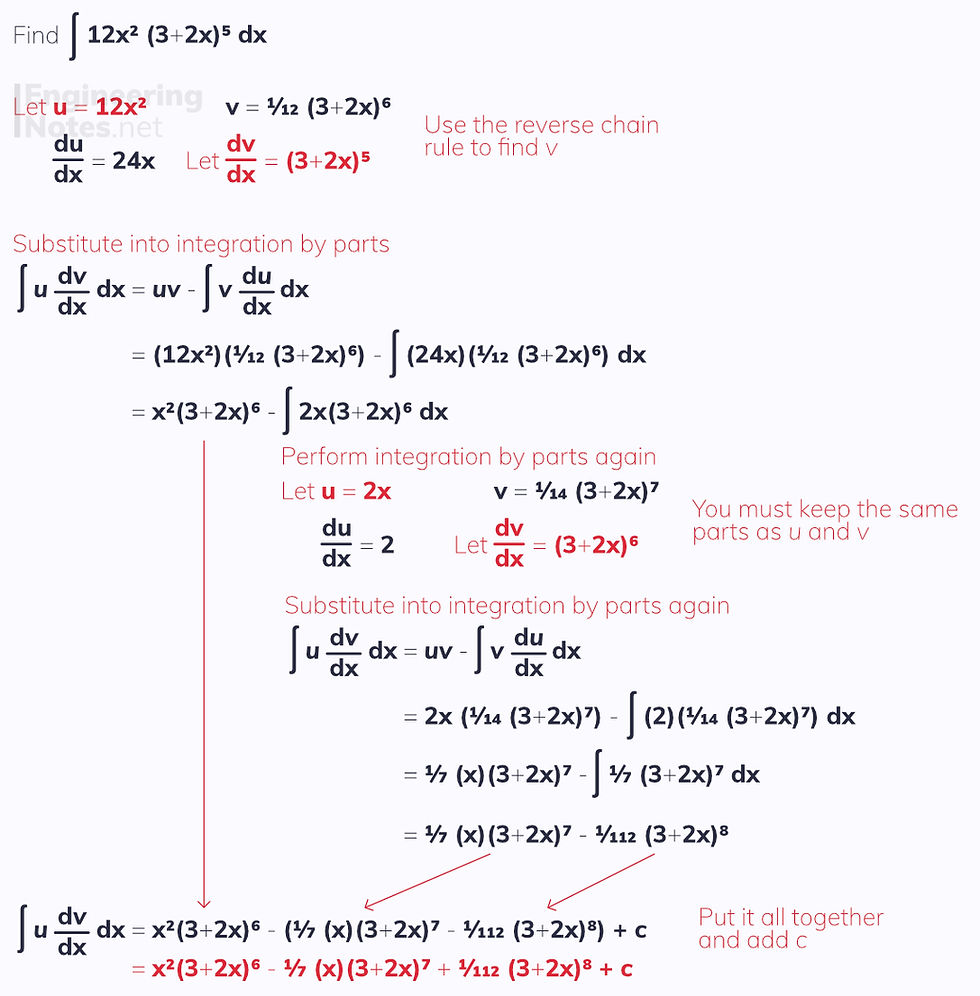
This is a fairly simple example, but they can get really very challenging. Often, you will end up with another integration by parts inside the first one - this is normal. If, however, you end up in never-ending circles of integration by parts, it means you have substituted the wrong part for v and u.

The Trapezium Rule

The trapezium rule is a numerical method used to approximate the area under a curve by dividing it into equally wide strips of varying heights:

The are n number of strips, so the width of each strip, h, is given as

h = (b - a)/n

The formula for the trapezium rule is derived from the area of a trapezium, ½(a+b)h, where a and b are the height of each dotted line (the parallel sides of the trapezium). The first is called y₀, then y₁, y₂, y₃ etc. until you get to the last one, yₙ:

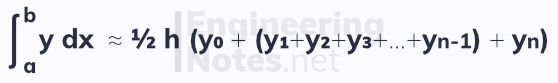
To use this, you need to make a table of all the y-values for each interval h of x. Then, input these into the formula. The greater n, the more accurate the approximation - however also the more time-consuming it is.

Differential Equations

Wow, you've made it through. Now for the bit where any of this is actually useful...

Separating Variables

This is the simplest method for solving differential equations, and is the only one we will cover under single maths. As the name suggests, it works y separating the variables:

When dy/dx = f(x) g(y), you can say ∫ 1/g(y) dy = ∫ f(x) dx

• Move all the y terms to the left where the dy is

• Move all the x terms to the right, including the dx

This allows you to integrate each side with respect to the variable on that side to solve the equation.

• You only need to add the ' +c ' to one side.

Just like when integrating an indefinite function, the initial result is a general solution and could be anywhere along the y-axis (see section on indefinite integral functions above). To fond the particular solution, you need to know a coordinate point on the curve - sometimes this is called a boundary condition.

Modelling with Differential Equations

A huge application of differentiation and integration is to model anything where the rate of change of something is dependent on the value of that something. For example, rabbit populations...