Sequences & Series
- A-Level Maths

- Sep 2, 2020
- 3 min read
Updated: Dec 7, 2020
A sequence is a list of numbers with a particular relation; a series is the sum of such a list of numbers. Sequences are sometimes a,so referred to as progressions.
Arithmetic
Arithmetic Sequences
An arithmetic sequence has a constant defined distance between terms, e.g. 1, 3, 5 7, 9 etc. The first term is 1 and the common difference is +2.
The common difference can be positive (the sequence is increasing) or negative (the series is decreasing).
To calculate the nth term, u(n), of an arithmetic sequence:

where a is the first term and d the common difference.
Arithmetic Series
An arithmetic series is the sum of all numbers in an arithmetic sequence. The sum of the first n terms is given by:

where a is the first term, d the common difference, and l the last term.
Geometric
Geometric Sequences
A geometric sequence is a sequence where there is a common ratio, not a common difference. This means the relationship between the numbers is a multiplication, not addition/subtraction. For example, the sequence 2, 4, 8, 16, 32 etc. is geometric - each term is multiplied by 2.
The formula for the nth term of a geometric sequence is:

where a is the first term and r is the common ratio.
Geometric Series
A geometric series is the sum of the first n terms of a geometric sequence. The sum of the first n terms is given by:

where a is the first term and r the common ratio.
The common ration cannot be 1, else each number would be the same and the sum is just n x a.
Sum to Infinity
As n tends towards infinity, the sum of the series is called the sum to infinity.
If a series is getting bigger, its sum tends to infinity, e.g. the series 2 + 4 + 8 + 16 + 32 etc. This happens when r > 1, and the series is known as divergent.
If a series is getting smaller, its sum tends to a finite value, e.g. the series 2 + 1 + 1/2 + 1/4 + 1/8 etc. This happens when r < 1, and the series is known as convergent.
When a series is convergent, we can calculate the fixed value that its sum tends to, its sum to infinity:

Sigma Notation
The Greek capital letter sigma, ∑, is used to note sums. Limits are shown above and below the ∑ to tell you from which term to which term to sum, followed by an expression. This is the function used to calculate every term in the sequence:

There are standard results for this you can substitute into other series:

If the series is given in the form of an expression, but there are too many terms to write out, just write out the first few to find the first term and common difference/ratio. Then use standard arithmetic/geometric sum equations.
Recurrence Relations
A recurrence relation defines each term of a sequence as a function of the previous term. This means you need to know at least one term in a sequence to work forwards or backwards from.
A recurrence relation is noted as:
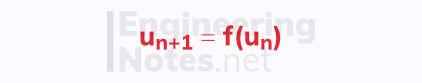
An example of how to calculate a sequence from a recurrence relation is:
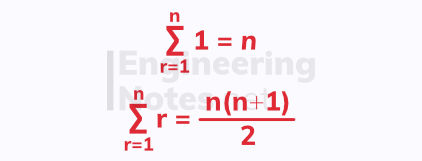
There are three forms a sequence can take, depending on the recurrence relation:
A sequence is increasing when u(n+1) > u(n)
A sequence is decreasing when u(n+1) < u(n)
A sequence is periodic if the terms repeat in a regular cycle: u(n+k) = u(n) where k is the order of the sequence.
A sequence can also take none of these three forms.
1, 3, 5, 7, 9... is increasing
6, 4, 2, 0, -2... is decreasing
1, 3, 5, 1, 3, 5, 1, 3, 5... is periodic with an order of 3
1, 7, 4, -9, 12... is none of the above.



Comments