Trigonometry

The graphs of sin, cos and tan are periodic - this means they repeat themselves for certain intervals.

• Both y = sinθ and y = cosθ have a repeating period of 360° or 2π

• Both y = sinθ and y = cosθ have a a maximum value of 1 and a minimum of -1

• y = sinθ and y = cosθ are the same shape, but offset by 90° or 1/2π.

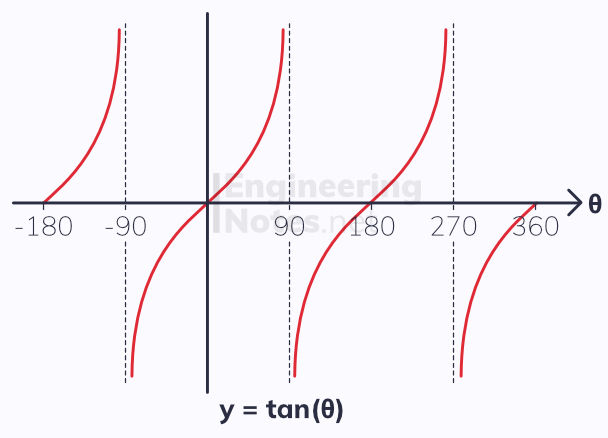
• y=tanθ or 1π. repeats every 180° or 1π.

• There are vertical asymptotes at 90° and then every 180° after in each direction. This means tanθ is undefined at these values.

Standard graph transformations apply to trigonometric graphs. See notes sheet on Functions, Graphs & Transformations

Sine & Cosine Rules

Often you will only know some side lengths or angles in a triangle, and will need to work out the missing measurements. When you know two side lengths and one of their corresponding (opposite) angles or vice versa, use the sine rule:

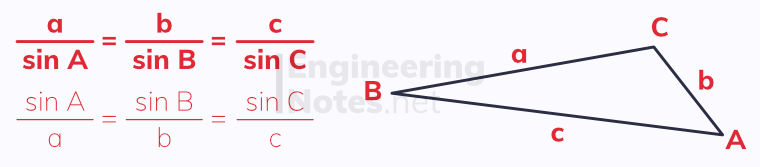
Sometimes the sine rule produces two possible solutions for a missing angle, an acute angle and an obtuse angle. Make sure you get the right one.

Cosine Rule

If you know just the three side lengths and no angles, use the cosine rule:

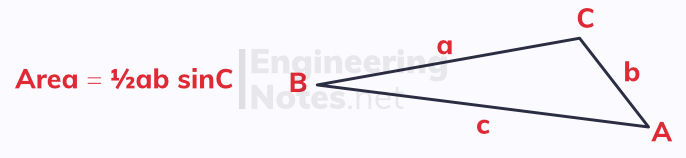
Area of a Triangle

To calculate the area of a triangle, you need to know two side lengths and the angle between these two sides:

CAST Diagrams & Quadrants

As well as their standard graphs, we can represent trig functions as circles in four quadrants. These diagrams are called CAST diagrams, and work by measuring an angle anticlockwise from the positive x-axis.

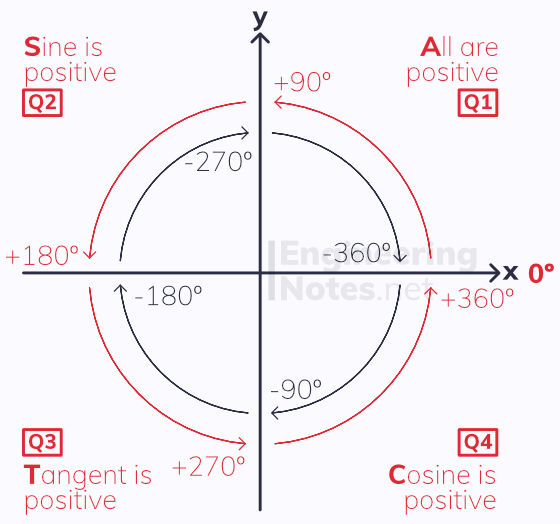
CAST is an acronym for which functions are positive in which quadrants (sections of the graph), starting in Q4 and going anticlockwise through Q4, Q1, Q2, Q3:

• In Q1, all of sinθ, cosθ and tanθ are all positive

• In Q2, only sinθ is positive

• In Q3, only tanθ is positive

• In Q4, only cosθ is positive

CAST diagram are useful for angles greater than 360°, as you can keep going round in circles and see which quadrant you end up in.

Radians

A radian is an alternative measure for angles. Typically, we use radians instead of degrees for angles because we can differentiate and integrate functions in radians, but not ones in degrees.

A radian is the angle subtended by a circular arc with a length equal to the radius of the circle:

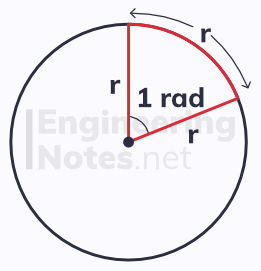
There are 2π in a circle, and half that in a semicircle. Therefore,

• to convert from degrees to radians, divide the angle in degrees by 180/π

• to convert from radians to degrees, multiply the angle in radians by 180/π

Arc Length

When using radians, calculating arc length is a lot easier than when using degrees:

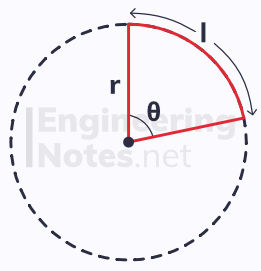
l = rθ arc length = radius x angle (when using radians)

The diagram above shows two arcs:

• the minor arc is the smaller one in red, with an angle θ

• the major arc is the larger dotted arc, with an angle of (2π-θ)

Sector Area

Again, this is a lot easier to calculate using radians.

A = 1/2 r²θ Sector Area = 1/2 x radius² x angle (when using radians)

Just like with arc, there are minor and major sectors.

Segment Area

Using sector area and the area of a triangle, you can easily work out the area of a segment:

• Work out the area of the sector using A = 1/2 r²θ

• Work out the area of the triangle inside using A = 1/2 ab sinC

• Subtract the area of the triangle from the sector area

Small Angle Approximations

When θ is small and measured in radians:

sinθ ≈ θ

tanθ ≈ θ

cosθ ≈ 1 - θ²/2

Trigonometric Ratios

There are a few exact values that are helpful to know off by heart for each of sine, cosine and tangent:

Sine

• sin 30° = sin π/6 = ½

• sin 60° = sin π/3 = (√3)/2

• sin 45° = sin π/4 = 1/√2 = (√2)/2

Cosine

• cos 30° = cos π/6 = (√3)/2

• cos 60° = cos π/3 = ½

• cos 45° = cos π/4 = 1/√2 = (√2)/2

Tangent

• tan 30° = tan π/6 = 1/√3 = (√3)/2

• tan 60° = tan π/3 = √3

• tan 45° = tan π/4 = 1

Sec, Cosec & Cot

Each of sine, cosine and tangent have their respective reciprocal functions: secant (sec), cosecant (cosec) and cotangent (cot):

Secant, sec

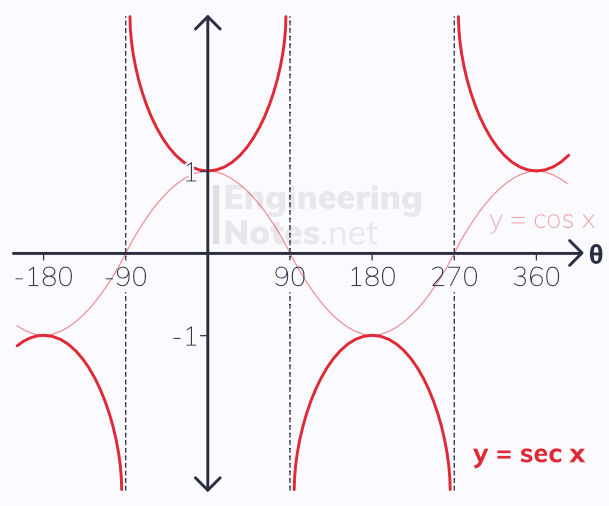
sec x = ⅟cos x

• sec x is undefined for values of x where cos x = 0, so has vertical asymptotes

• y = sec x is symmetrical about the y-axis

• y = sec x has a period of 360° or 2π radians

• the range of y = sec x is y ≤ -1 and y ≥ 1

Cosecant, cosec

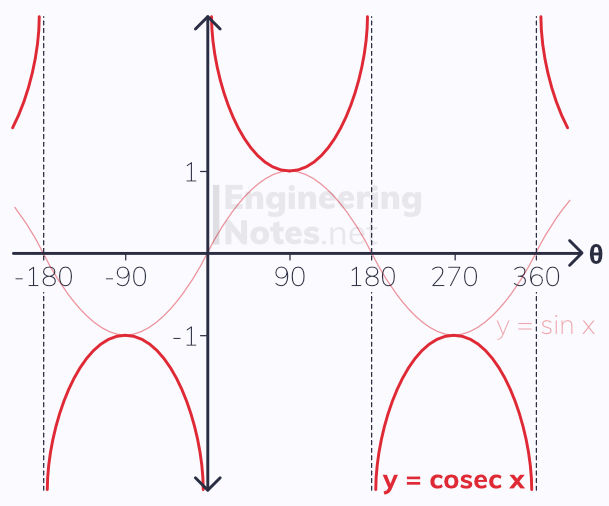
cosec x = ⅟sin x

• cosec x is undefined for values of x where sin x = 0, so has vertical asymptotes

• y = sec x has a period of 360° or 2π radians

• the range of y = sec x is y ≤ -1 and y ≥ 1

Cotangent, cot

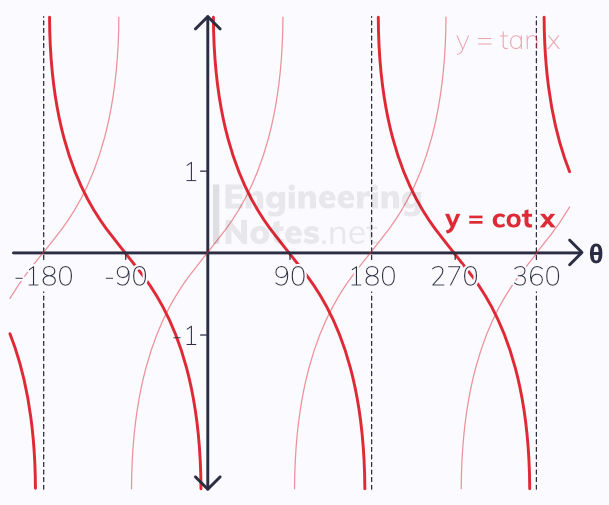
cot x = ⅟tan x

• cot x is undefined for values of x where tan x = 0

• y = sec x has a period of 180° or 1π radians

• the range of y = sec x is y ∈ ℝ

Trigonometric Identities

For all values of θ, whether in radians or degrees,

sin² θ + cos² θ ≡ 1

tan θ ≡ sin θ / cos θ

You can use these identities to solve trigonometric equations. However, it is important to note a few things:

• sin θ = k and cos θ = k only have solutions when -1 ≤ k ≤ 1

• tan θ = k for all values of k

• when using a calculator to find sin‾¹, cos‾¹ or tan‾¹, the calculator will give the principle values only - this will probably not be all the values you need

cot x = cos x / sin x

1 + tan² x ≡ sec² x

1 + cot² x ≡ cosec² x

Again, there are a few things to note:

• sec x = k and cosec x = k have no solutions when -1 < k <1

• cot x = k for all values of k

Angle Addition Formulae

sin(A ± B) ≡ sinA cosB ± cosA sinB

cos(A ± B) ≡ cosA cos B ∓ sinA sinB

tan(A ± B) ≡ (tanA ± tanB) / (1 ∓ tanA tanB)

These are sometimes also referred to as the compound-angle formulae. They work for degrees and radians.

Double Angle Formulae

These are derived from the angle addition formulae above.

sin2A ≡ 2 sinA cosA

cos2A ≡ cos²A - sin²A ≡ 2cos²A - 1 ≡ 1 - 2sin²A

tan2A ≡ (2 tanA) / (1 - tan²A)

The R-Addition Formula

Trigonometric expressions in the form a sinx ± b sinx or a cosx ± b sinx, where a and b are positive, can be simplified:

• a sinx ± b sinx can be expressed as R sin(x ± α)

• a cosx ± b sinx can be expressed as R cos(x ∓ α)

Where R > 0 and 0° < α < 90° (or ½π) and:

R cos α = a

R sin α = b

R = √(a² + b²)

Inverse Trigonometric Functions

Sine, cosine and tangent all have inverse functions, known as arcsin, arccos and arctan.

Arcsin & Arccos

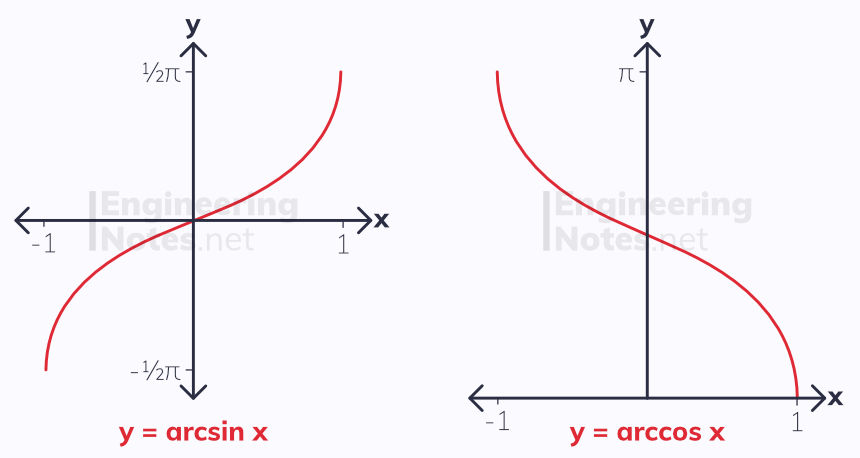
• The domain of y = arcsinx and y = arccosx is -1 ≤ x ≤ 1

• The range of y = arcsinx is -½π ≤ arcsinx ≤ ½π or -90° ≤ arcsinx ≤ 90°

• The range of y = arccosx is 0 ≤ arccosx ≤ π or 0° ≤ arccosx ≤ 180°

Arctan

• The domain of y = arctanx is x ∈ ℝ

• The range of y = arctanx is -½π ≤ arctanx ≤ ½π or -90° ≤ arctanx ≤ 90°